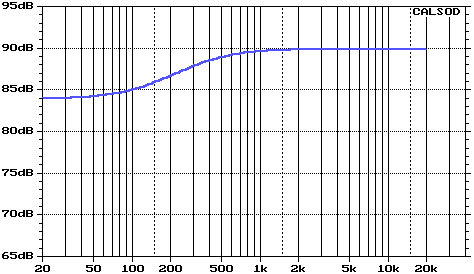
An important loudspeaker design consideration is the baffle diffraction step, which is a result of the transition in the acoustical load presented to the loudspeaker. At high frequencies, a loudspeaker tends to project sound only in the forward direction that is, it has a 2 pi hemispherical radiation. At low frequencies, a loudspeaker undergoes a transition by which it begins to radiate sound in all directions, or a 4 pi spherical radiation. The result is an apparent reduction of bass response because the low frequency energy density is reduced in the forward direction where the listener is located. The overall effect is a gradual 6 dB shelving action at low frequencies, such that the bass is 6dB lower in level than the treble. See Figure 1 for a graph of baffle diffraction loss for an arbitrary 18" wide baffle.

Figure 1. Effects of baffle diffraction for 18" wide baffle
Please note that this diffraction loss curve is for an
ideal loudspeaker mounted in a sphere, which exhibits a very smooth transition. Other
shapes, such as the common rectangular enclosure, will introduce peaks and dips in the
response, but the overall trend remains the same. Very little may be done to predict these
response anomalies as they are predominantly spurious. The only reliable way to determine
whether these peaks and dips are objectionable is with listening tests and acoustic
measurements.
Baffle diffraction loss is a function of wavelength. The wavelength (l) of a given
frequency at room temperature and standard atmospheric pressure may be found by the
following equation:
![]()
The baffle diffraction step may be most accurately described as a loss of bass, and not a gain in treble. However, if we view a graph of baffle diffraction, the amplitude "rises" as we move from left to right across the frequency axis. As an approximation, the rise begins at the frequency whose wavelength is 1/8 the smallest dimension of the baffle. This dimension is typically the width of the loudspeaker since most are tall and narrow. Using the same 18" baffle as in the previous example, the response would begin to rise at [1/8 * (13560/18)], or 94 Hz. Also, the maximum amplitude is attained at a frequency whose wavelength is twice the smallest dimension of the baffle; in this case [2(13560/18)], or 1.5 kHz. The -3dB point has been empirically determined to be:
![]()
The f3 for our
arbitrary baffle width of 18" would be 253 Hz. All these calculations are in
agreement with the baffle diffraction loss simulation depicted in Figure 1. Based on the
above equation, it becomes clear that diffraction loss begins at higher frequencies as the
baffle width is decreased. Conversely, for an infinitely large baffle, there will be no
loss whatsoever as the loudspeaker is radiating into a 2 pi environment at all
frequencies. This effect may be approximated by mounting the loudspeaker within the plane
of a large wall.
The baffle diffraction step is generally viewed as a problem since it results in a
non-flat frequency response. However, a mirror-imaged shelving network may be implemented
to correct the frequency response to a flat amplitude. All that is needed is a network
that attenuates high frequencies at a rate of 6 dB/octave, and "topping out" at
6 dB's of overall attenuation. Dr. John Murphy has documented an approach for compensating
for the baffle step, so it will not be repeated here.
I must say, however, that it is preferable to use an iterative process to develop a
compensation circuit. This capability is offered by CALSOD and other such crossover
optimization programs, after which the results should be verified with acoustic
measurements. It is often possible to devise a compensation circuit that also incorporates
filtering duties, rather than implementing a dedicated circuit. A dedicated circuit may
introduce unnecessary series inductors into the circuit. These inductors are typically
expensive, particularly if low DCR types are employed -- necessary to minimize performance
degradation. Furthermore, inductor placement becomes more troublesome if mutual
cross-coupling is to be avoided. However, those who do not possess sophisticated
software/hardware will certainly find the dedicated circuit to be vastly superior to
simply ignoring the phenomenon.
Most (but not all) loudspeaker manufacturers publish response curves taken in a 2 pi
environment. Therefore, the effects of baffle diffraction loss are not depicted.
Consequently, if the frequency response curve indicates flat response, there will be a
reduction in the bass response when the driver is placed in an enclosure and into a room,
a 4 pi environment. This is a very important concept to understand since it will affect
the crossover design and also the maximum sensitivity attainable with the particular
driver.
For example, suppose you have a woofer rated at 91dB/2.83V/1m and a tweeter also rated at
91dB/2.83V/1m. At first glance they would seem to be a perfect match for one another, at
least in terms of sensitivity. However, baffle loss has not been factored in. In order to
achieve flat amplitude response down to the low frequency limits of the driver, the
midrange frequencies must be shelved down by 6dB, resulting in an 85dB sensitive woofer.
This of course would also
necessitate padding down the tweeter to mate with the woofer. In reality, it isn't
necessary to shelve down the entire 6dB if a somewhat premature rolloff in the upper bass
is acceptable, whereby allowing for a higher average sensitivity throughout the midband.
This decision is a purely subjective design choice that should ultimately be based on the
specific circumstances and the listener's preferences. Many commercially designed
loudspeakers opt for this extra sensitivity and typically choose a 3-4dB shelf. Here
again, an iterative optimization program is useful for determining a crossover network
which will provide this response.
No fancy ending to this story, but I do hope this information is somewhat helpful in
explaining an esoteric and often misunderstood area of loudspeaker design. This
information is an excerpt from an electrostatic loudspeaker paper I've written, and
modified to cover a couple of issues not in the original copy. I know this overview is
somewhat intimidating to a nontechnically-minded person, but I struggle for a way to
simplify without also leaving out useful information. Good luck to all.